Acceptable
Level of Risk and Phytosanitary Measures
Ricardo Sgrillo
CEPEC/CEPLAC
Rod. Ilheus-Itabuna, km 22
45650-000 Ilheus, BA, Brazil
This paper represents the views of
the author and not necessarily those of his country and/or organization.
Introduction
The
concepts of Appropriate Level of Protection (ALP) and Acceptable
Level of Risk (ALR) are important topics of discussion in national
and international organizations concerned with the harmonization of sanitary
and phytosanitary measures.The purpose
of this paper is to contribute to these discussions with one explanation
of these concepts as they may be interpreted for the establishment of phytosanitary
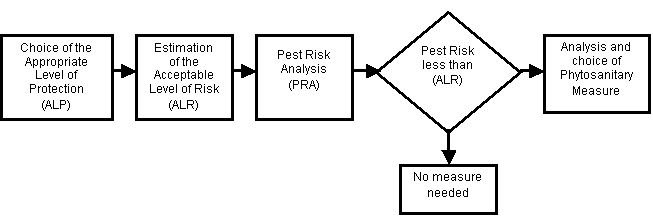
measures as illustrated in Figure 1:
Figure 1.Process for the establishment of phytosanitary measures.

1.Appropriate
Level of Protection
The
first box in Figure 1 represents the choice of the appropriate level
of protection, a sovereign right of each country. For phytosanitary
purposes, the appropriate level of protection is the level of protection
that a country decides is necessary to protect plant health and life against
the harmful effects of plant pests.This
level of protection is achieved by reducing the risk of pest establishment
to specified levels, the acceptable level of risk.
To
stipulate a level of protection for quarantine pests, it is first necessary
to relate this to the pest risk, which in turn is related to the probability
of pest establishment.This raises
two key questions:
(1)
How many pests are required to establish; and,
(2)
What prevalence of pests will be allowed in a lot, consignment, year, or
some other unit measurement?
The
answer to the first question depends on many conditions, including biological
and other factors, but the intuitive answer to the second question is “zero”.No
country wants to accept shipments that are contaminated with pests.However,
according to the principle of managed risk, “zero” risk is not possible.Even
the prohibition of trade is not a zero-risk option (prohibition may actually
increase risk because it can increase the motivation for smuggling).
Recognizing
that zero risk is not an option and also that some level of pest prevalence
is required for pest introduction, countries desire a level “close to zero”.And
what is the meaning of "as close to zero as possible"?To
discuss these points, it is important to first understand the important
role of probability analysis.
Two
key concepts need to be defined at the outset:
unit
risk:the
probability of introduction of a quarantine pest through the importation
of one unit of the product during a specific period of time.
product
risk:the
probability of introduction of a quarantine pest through the importation
of all units of one product, from one origin, during a specific period
of time.
The
importation risk is estimated by multiplying the unit risk
by the number of all the units of the product.The
result represents the number of expected introductions per time unit.The
reciprocal of the importation risk (1/risk) expresses the expected period
of time between two introductions, which may be called the introduction
period.Each case may be described
by the unit introduction period
and product introduction period.Using
one year as the time unit, and assuming a risk of 0.01, we would expect
one introduction each 100 years (1/0.01).Table
1 shows some of the relationships between unit risk, product
risk and correspondent introduction periods where there are
100,000 total units in the consignments in one year.
Table
1. Relationship
between unit risk, product risk and corresponding introduction
periods.
|
|
(Years) |
|
|
|
1 |
1 |
100,000 |
(one introduction each 5.2 minutes) |
|
0.1 |
10 |
10,000 |
(one introduction each 52 minutes) |
|
0.01 |
100 |
1,000 |
(one introduction each 8.7 hours) |
|
0.001 |
1000 |
100 |
(one introduction each 3,6 days) |
|
0.0001 |
10,000 |
10 |
(one introduction each 36.5 days) |
|
0.00001 |
100,000 |
1 |
(one introduction each year) |
|
0.000001 |
1,000,000 |
0,1 |
(one introduction each 10 years) |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
1
Number of expected introductions in one year, considering the importation
of 100,000 units
Each
row in Table 1 represents a different level of product risk and a corresponding
introduction period which also represents a level of protection.Some
conclusions that may be drawn from the Table include:
§The
level of protection is inversely proportional to the risk but it
is not the risk itself.
§Zero
risk (or full protection) is not practically achievable because there is
always some likelihood of pest introduction if the quarantine pest is present
in the origin.
§More
protection is attained with lower risk.
§The
risk of introduction increases with the increasing number of imports, implying
that a country could perhaps require an annual quota for importation to
maintain an acceptable level of risk (although technically justified, the
practical implications of implementing such a requirement may be difficult).
So
what is the meaning of "close to zero"?Is
0.00001 or the probability one introduction each 100,000 years close to
zero?Or is it the probability of
one introduction every 500 years?The
main point to note at this stage is that although countries have the sovereign
right to choose their appropriate level of protection, it is difficult
to understand what this means unless it is expressed quantitatively in
relation to an acceptable level of risk.This
is consistent with the principle of transparency.
One
way to express the level of protection numerically is as log10
of the product introduction period[1]:
![]() Equation
1
Equation
1
Table
2 demonstrates the relationship of the product introduction period
and product risk to the level of protection associated with
representative levels from -1 to 6.As
an example, a country may decide that 3 is an appropriate level of protection.This
means that it accepts a product risk of 0.001, which is equivalent
to one introduction every 1,000 years.This
does not mean there will be an introduction every 1,000 years, but rather
that this is the mean of a probability distribution with some probability
that an introduction will occur in the first year and likewise a probability
that introduction will occur in 999 years or later.
Table
2.Level
of Protection in relation to product introduction period and
product risk
|
|
|
(introductions per time unit) |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
The decision to specify an appropriate level of protection may not
be based only on biological criteria but could also account for political,
social, and economic aspects.For
example:
·The
country produces less than is needed and therefore has to import to supply
the market (pushes ALP down)
·The
country will lose international markets through the establishment of the
pest (pushes ALP up)
·The
importation would lower the price of the product, reducing the demand pressure
and helping to reduce inflation pressure (pushes ALP down)
·The
establishment of the pest will increase the production costs and the product
would loose competitiveness (pushes ALP down)
·The
national industry will have to improve the competitiveness of its product
by improving the quality and decreasing the price (pushes ALP down)
·New
employment would be generated through the need for handling a greater volume
of the product (pushes ALP down).
· The
crop in the importing country has strategic economic and social importance
and the establishment of a new pest would have catastrophic consequences
(strongly pushes ALP up)
2. Acceptable
Level of Risk
The
second box in Figure 1 refers to the estimation of the acceptable level
of risk.This should be a straightforward
procedure.From Equation 1 we can
estimate the acceptable level of risk based on the appropriate
level of protection and from the number of units to be imported:
 Equation
2
Equation
2
For
example, if a country establishes its appropriate level of protection
as 2.7 (one introduction each 500 years), and intends to import 2,000 units,
then the acceptable level of risk is 0.000001.This
ALR refers to the unit risk.
3.Pest
Risk Analysis
The
next box in Figure 1 refers to pest risk analysis.This
is a well-known procedure that does not require discussion in this paper.It
is only necessary to note that a quantitative PRA would generate a probability
of introduction and the statistics associated with it.If
this probability is less then the acceptable level of risk, no measure
should be required.
4.Phytosanitary
Measure
The
last box in Figure 1 represents the analysis and choice of phytosanitary
measures.This is also a straightforward
procedure but it requires that two additional concepts are defined:
risk
of failure (of the measure):the
proportion of the pest population that is not removed from the product
by the phytosanitary measure.
efficacy
(of the measure):the
percentage of the population that is removed from the product by the phytosanitary
measure.Sampling
and experimentation are used to estimate efficacy.
The
relationship between these variables is expressed in Equation 3:
![]() Equation
3
Equation
3
The
objective is to find a measure whose risk of failure multiplied
by the probability of introduction (as estimated by PRA) will be
equal or lower than the acceptable level of risk.This
may be called the minimum required efficacy and the corresponding
risk of failure would be the maximum allowable risk of failure.These
concepts, together with Equation 3 are used to generate equation 4, where
the minimum required efficacy may be estimated from the acceptable
level of risk and from the probability of introduction:
![]() Equation
4
Equation
4
![]()
After
this point it is necessary to compare the minimum required efficacy
(or the maximum allowable risk of failure) with the actual efficacies
(or risk of failure) of the available phytosanitary measures and
to choose the most appropriate measure on this basis, considering also
the need to choose the least trade restrictive measure if there are equivalent
options.An economic analysis may
also be useful for further evaluating measures where there are multiple
options.
5. Example
To
demonstrate the application of the above concepts in practice, assume a
hypothetical situation where:
§Country
A wants to export 20,000 boxes/year of a product to country B.
§Country
B
is concerned with a quarantine pest that occurs in country A
and is known to be associated with the product to be exported.
The
task is to choose appropriate measures based on a specified level of protection
and calculations made from data supplied through PRA.
5.1.
Appropriate Level of Protection (ALP) for country B:
Taking
into account relevant economic, social and political factors, country B
stipulates an appropriated level of protection of 2.48.The
expected period of time between two introductions is 300 years and this
is considered satisfactory for country B.
5.2.
Acceptable Level of Risk (ALR)
The
acceptable
level of risk, estimated with Equation 2 is:
![]()
5.3.
Pest Risk Analysis
Country
B
developed a quantitative PRA with the results found in Table 3.
Table
3. Results
of the quantitative PRA
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Note:
the pest risk analysis should be complete, evaluating also the probability
of establishment, economic losses, etcetera.All
relevant statistics should also be estimated and considered (e.g., distribution
of errors).
5.4
Choice of Phytosanitary Measure
To
begin, country B uses Equation 4 to estimate the minimum required
efficacy and the maximum allowable risk of failure:
![]()
![]()
To
facilitate the choice of the measures, country B will compare the
measures through a matrix representing the relationship of the maximum
allowed risk of failure and the risk of failure of each
measure (Table 4). The threshold value is 1.Any
measure with a value smaller then 1 does not provide for the appropriate
level of protection and vice-versa.Other
methods to compare measures could be used.
Table
4. Parameters
of the available measures
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Risk
(probability) of failure of the measure |
1 |
0.1 |
0.000002 |
0.008 |
0.002 |
0.000001 |
0 |
|
|
|
|
|
|
|
|
|
1
Efficacy data is derived from experimentation and sampling (confidence
limits should also be considered)
2
Measure index = ![]()
Figure
2. Graphical
comparison of the measures
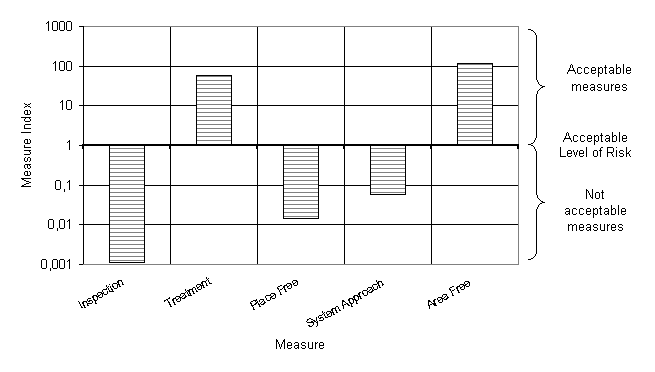
5.5.
Conclusions
CountryB
may accept the import of 20,000 boxes from country A, but should
require treatment to achieve the appropriate level of protection.Area
freedom would also be acceptable since this would provide even a greater
protection level, but it may be viewed as more trade restrictive.
5.6
Alternatives
Suppose that the exporting country has no free areas and that there is no treatment for the pest.The only thing that the exporting country could offer as a phytosanitary measure in this case is a systems approach with the efficiency shown in Table 4.
Following
all the steps backward, country B estimates that the levelof
protection it will achieve would correspond to one introduction every
15 years.However, the appropriate
level of protection could be attained with the system approach if country
B authorizes importation of only 1,000 boxes each year.Also
if country A increases the efficacy of its systems approach
up to the minimum required efficicacy (99.98847) the appropriate
level of protection established by country B will be achieved.
6. Summary
of the concepts and definitions introduced
Following
are the main concepts introduced in this paper and definitions proposed
for each.
|
Efficacy
(of a phytosanitary measure)
|
|
|
Level
of protection
|
Log10
of the product introduction period
|
|
Maximum
allowable risk of failure (MAR)
|
The
maximum level of failure for a phytosanitary measure which achieves the
acceptable level of risk
|
|
Minimum
required efficacy (MRE)
|
Minimum efficacy of
a phytosanitary measure required to achieve the acceptable level of risk
|
|
Product
introduction period
|
Expected
period of time between the mean probability of two introductions resulting
from the importation of all units of one product from one origin. Mathematically,
the reciprocal of the product risk
|
|
Product
risk
|
Probability
of introduction of a pest through the importation of all units of one product,
from one origin, during a specified period of time
|
|
Risk
of failure (of a phytosanitary measure)
|
Proportion
of a pest population that is not removed from a product by a phytosanitary
measure.
|
|
Unit
introduction period
|
Expected
period of time between two introductions resulting from the importation
of one unit of the product. Mathematically, the reciprocal of the unit
risk
|
|
Unit
risk
|
Probability
of introduction of a pest through the importation of one unit of the product
during a specific period of time
|
7.Graphical
Summary of the Relationships
Figure
3 is a graphical demonstration of the relationship between the maximum
allowable risk of failure of the phytosanitary measure, the probability
of introduction (as estimated by PRA), and the appropriate level
of protection.
Figure
3.Relationship
between the risk of failure, probability of introduction and appropriate
level of protection
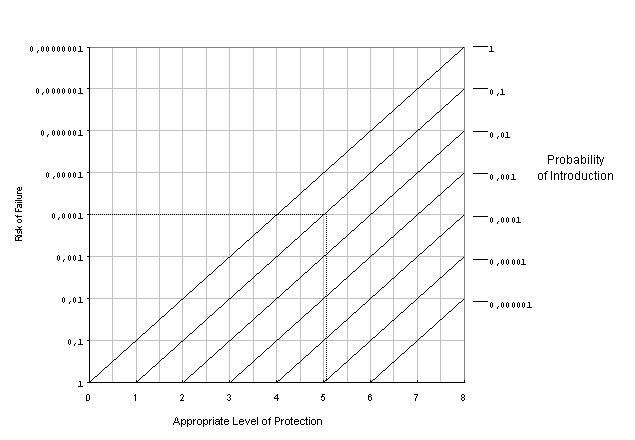
The
dotted line in the graph is to show that for an appropriate level of
protection equal to 5 and a probability of introduction equal
to 0.1, it would be necessary to have a phytosanitary measure with a risk
of failure less than 0.0001 (or efficacy greater then 99.99 %).
Note:
as this graph was constructed based on one unit of the product, the risk
of failure must
be divided by the total number of units to be imported. Many thanks to Robert Griffin (USDA) for the final
revision.